|
- Seismic Waves
- Earthquake Magntiude
- Differences between Earthquake Magnitudes
- Application of Magnitudes
Written by: WOO Wang-chun September 2012
The earthquake magnitude quantifies the size or scale of an earthquake, which is generally related to the energy released. The concept was first proposed by Mr Charles Richter in 1935. After decades of development, there are now dozens of methods to compute earthquake magnitudes. Four of them are most commonly used, which are local magnitude Ml, body wave magnitude mB, surface wave magnitude Ms and moment magnitude Mw. There are also some more specialised methods, such as duration magnitude Md based on the duration of shaking, macroseismic magnitude Mms based on felt and damage reports, tsunami magnitude Mt based on the height of the tsunami generated etc..
- Seismic Waves
After an earthquake occurs, the energy released will propagate outwards in all directions in the form of seismic waves. Generally speaking, the amplitude of seismic waves recorded by a seismograph is higher for a larger earthquake. As seismic waves attenuate while spreading out, the seismic wave recorded at places farther from the epicentre is smaller. One can compute the earthquake magnitude based on the amplitude of the seismic waves recorded by a seismograph, after accounting for the distance between the earthquake epicentre and the seismograph station (epicentral distance).
Seismic waves are classified into two broad categories. One propagates within the body of the Earth, thus known as "body waves", which can be further divided into longitudinal wave P and transverse wave S. The other propagates along the surface of the Earth, thus known as "surface waves". The speeds of propagation of different seismic waves are different. P waves travel the fastest, followed by S waves and surface waves. Nevertheless, surface waves are the largest and thus inflicted the greatest damages, whereas P waves are the smallest. The epicentral distance can be computed with the arrival time of different waves.
 Back to content Back to content
- Earthquake Magntiude
The local magnitude Ml is applicable to an earthquake near a seismograph station, and is commonly known as the "Richter Scale". The body wave magnitude mB, surface wave magnitude Ms and moment magnitude Mw, commonly adopted by most earthquake and tsunami monitoring centres nowadays, are strictly speaking not "Richter Scale". The Observatory no longer describes magnitudes as on "Richter Scale" after enhancements of earthquake information services in May 2011.
(1) Local Magnitude Ml
The local magnitude Ml is best illustrated with the following example:
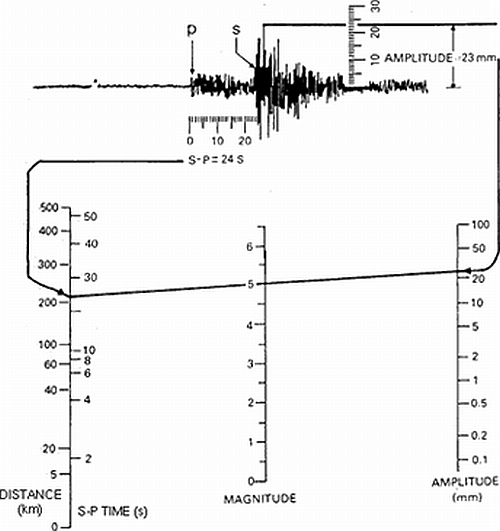
Fig.1 (Source: United States Geological Survey)
In the diagram, the difference in the time of arrival between the transverse wave S and the longitudinal wave P (S - P) is about 24 seconds. According to the scale on the left, the epicentral distance is around 210 km. The amplitude of the surface wave is measured to be 23 mm on the chart. A straight line joining the 210 km on the left and the 23 mm on the right would intersect with the scale marked as MAGNITUDE at 5.0, which is the Ml of this earthquake.
In local magnitudes Ml, a magnitude zero earthquake is defined as one that induces 1 m of amplitude on a standard seismometer located at 100 km from the epicentre. Its relationship with the maximum amplitude Amax is depicted as follow:
Ml = log Amax - log A0
where - log A0 is the term to adjust for the distance, as tabled in "Elementary Seismology (1958)" by Richter. A standard seismometer is a Wood-Anderson (WA) horizontal component torsion seismometer. Seismographs recorded by other kinds of seismometers would have to be converted to a "synthesised WA seismograph" before used for computing local magnitude Ml.
As shown from the formula, amplitude increases with Ml logarithmically. An increase of amplitude Amax by ten times corresponds to an increase of Ml by 1.0. Because increase of Amax by ten times is equivalent to an increase in energy released by 32 times, every increment of 1.0 in Ml means an increase in energy released by 32 times. For example, the energy released by a Ml 7.8 earthquake is 32 times that of a Ml 6.8 earthquake, or 128 times (i.e. 32(7.8-6.4)) that of an Ml 6.4 earthquake.
(2) Body Wave Magnitude mB
The periods of body waves generally fall between 0.5 second and 12 seconds. Seismic waves within this range of periods can be used to compute body wave magnitude mB. Its formula is similar to local magnitude Ml, as follow:
mB = log(A/T)max + Q(Δ,h)
where A and T are body wave amplitude and period respectively that can be obtained by measuring the seismic waves. Q(Δ,h) is the distance adjustment term, related to the epicentral distance Δ and the hypocentral depth h. It can be obtained through table lookup.
(3) Surface Wave Magnitude Ms
The periods of surface waves are generally 20 ± 2 seconds. Seismic waves around this period can be used to compute the surface wave magnitude Ms. Its formula is similar to those of local magnitude Ml and body wave magnitude mB, as follow:
Ms = log(A/T)max + 1.66 log Δ + 3.3
where A and T are surface wave amplitude and period respectively that can be obtained by measuring the seismic waves. Δ is the epicentral distance. It is worth pointing out that when computing Ms only stations with epicentral distances of 2? < Δ < 160? (equivalent to 220 km < Δ < 17,800 km on great circle distance) can be used, i.e. stations too close are not usable.
(4) Moment Magnitude Mw
Unlike local magnitude Ml, body wave magnitude mB and surface wave magnitude Ms, the definition of the moment magnitude Mw is not directly tied in with seismic waves. Its formula is as follow:
Mw = 2/3 log M0 - 6.06
where M0 is the seismic moment, equal to the product of shear modulus , area of the rupture A, and average displacement d.
As it is related to the physical process of an earthquake, moment magnitude Mw is a better representation of the energy released. It however has a much higher complexity of measurement and computation and thus takes longer time to compute. In recent years, scientists developed moment magnitude Mwp based on P-wave applicable to earthquakes of magnitude 5.5 or above. As Mwp is based on P-wave, the fastest seismic wave, it only takes dozens of minutes to compute, shorter than several hours normally required to compute Mw accurately.
 Back to content Back to content
- Differences between Earthquake Magnitudes
It is normal for an earthquake to have different magnitudes on various magnitude scales. Sometimes the difference could be quite large. For example, in the Deep Bay earthquake on 19 Nov 2010, the surface wave magnitude was 2.8 while the local wave magnitude was 3.7, differing almost by one.
The following chart shows the approximate relationships between historical earthquakes:
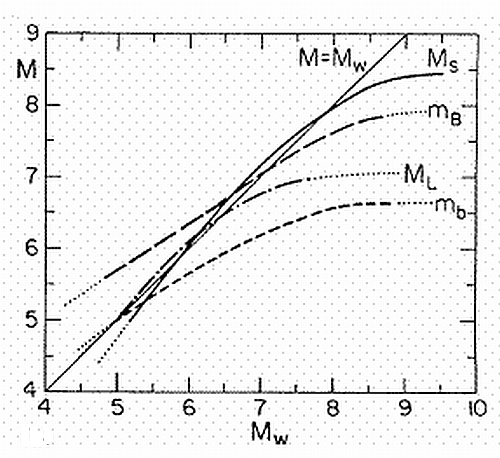
Fig.2 Relationship between different magnitude scales. M L on the chart is Ml in this text, m B is mB in this text and m b is a variant of mB. (Source: Technophysics, 93, No. 3/4 Kanamori, Magnitude scale and quantification of earthquakes, 1983, Fig 4, p. 193)
As shown in the chart, local magnitude Ml, body wave magnitude mB and surface wave magnitude Ms would saturate, i.e. beyond some thresholds a larger earthquake would not have higher magnitudes. For example, in the Alaska earthquake in 1964, the moment magnitude Mw was 9.1 while the surface wave magnitude Ms was 8.4 only. The body wave magnitude mB was even lower at 6.2. As another example, in the Tohoku Japan earthquake in 2011, the moment magnitude Mw was 9.0, while the surface wave magnitude Ms and the body wave magnitude mB were only 8.3 and 7.2 respectively.
Generally speaking, the local magnitude Ml saturates at around 7, while body wave magnitude mB saturates at around 8, and surface wave magnitude Ms saturates at around 8.5.
 Back to content Back to content
- Application of Magnitudes
Since body wave travels the fastest, the body wave magnitude mB is normally the earliest available earthquake magnitude. It is thus commonly adopted in quick earthquake messages. As it would saturate around magnitude 8, however, body wave magnitude mB is not the most suitable for large earthquakes. Due to slow travelling speed of surface waves, surface wave magnitude Ms is often only available after several tens of minutes. But its saturation point is higher and thus more suitable for larger earthquakes. Moment magnitude Mw would not saturate and is in theory the best one for large earthquakes. It however takes even longer time to compute than mB or Ms.
It is commonly observed that, after a large earthquake, earthquake and tsunami monitoring centres would first promulgate a magnitude but only to revise it upward dozens of minutes or couple of hours later. One of the major reasons is that the first calculated magnitude is a saturated mB, being updated after Ms with higher saturation point or Mw that would not saturate become available.
Following the emergence of P-wave moment magntidue Mwp, some earthquake and tsunami monitoring centres including the Hong Kong Observatory have started experimental uses of Mwp. When the technology matures, it would help monitoring centres determine the magnitudes of large earthquakes more rapidly and accurately.
 Back to content Back to content
|


